SetMatrixZero

Set matrix zero

Question

Given a matrix, if an element is 0, set its entire row and column to 0. Do it in place.

Question Link : Set Matrix Zero

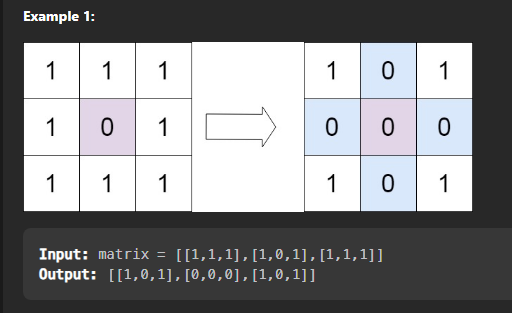
Let's discuss about the problem , what it demands and how to approach the problem.

// my approach

🎱 Step 1 : first find the place where zero lies in the matrix and store them in a set to avoid duplication of the position . Maintain two sets one for row and one for col.

🎱 Step 2 : Now itereate through the matrix and then simply check if either the row or col any of them is present in the set , if yes then set the value of that position to zero.

Code :

class Solution {
    public: 
    void setZeroes(vector<vector<int>>& matrix) {
        int n = matrix.size();
        int m = matrix[0].size();
        set<int> row;
        set<int> col;
        for(int i = 0 ; i < n ; i++){
            for(int j = 0 ; j < m ; j++){
                if(matrix[i][j] == 0){
                    row.insert(i);
                    col.insert(j);
                }
            }
        }
        for(int i = 0 ; i < n ; i++){
            for(int j = 0 ; j < m ; j++){
                if(row.count(i) || col.count(j)){
                    matrix[i][j] = 0;
                }    
            }
        }
    }
};

• Time Complexity : O(n*m)
• Space Complexity : O(n+m)

Another approach to optimize the space used :

What we can do is that instead of storing the rows and cols in a set , we can mark the row and cols in which the zero is present by setting the value of the first element of that row and col to zero.

Now we can iterate through the matrix and check if the first element of that row or col is zero , if yes then set the value of that position to zero.

Optimized Code :

class Solution {
    public: 

    void setZeroes(vector<vector<int>>& matrix) {
        int n = matrix.size();
        int m = matrix[0].size();
        bool row = false;
        bool col = false;
        for(int i = 0 ; i < n ; i++){
            for(int j = 0 ; j < m ; j++){
                if(matrix[i][j] == 0){
                    if(i == 0) row = true;
                    if(j == 0) col = true;
                    matrix[0][j] = 0;
                    matrix[i][0] = 0;
                }
            }
        }
        for(int i = 1 ; i < n ; i++){
            for(int j = 1 ; j < m ; j++){
                if(matrix[i][0] == 0 || matrix[0][j] == 0){
                    matrix[i][j] = 0;
                }
            }
        }
        if(row){
            for(int i = 0 ; i < m ; i++){
                matrix[0][i] = 0;
            }
        }
        if(col){
            for(int i = 0 ; i < n ; i++){
                matrix[i][0] = 0;
            }
        }
    }
};
// Time Complexity : O(n*m) + O(n*m)
// Space Complexity : O(1) -